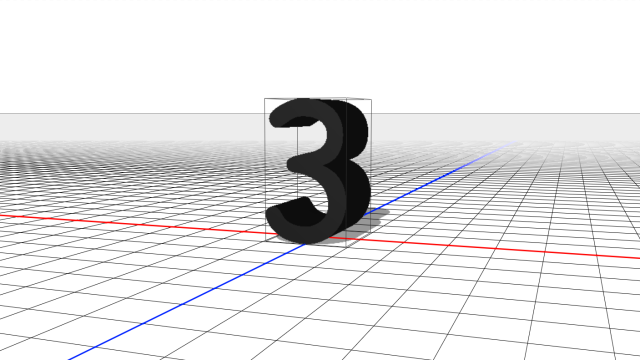
Just on the heels of finding three cubed numbers that sum to 42, scientists have passed another important milestone by finding three enormous cubes that sum to 3.
After finding three-cubes solutions for each integer less than 100, mathematicians set their sites on another milestone: finding another sum-of-three-cubes solution for the number 3. As simple as it sounds, it’s something researchers have been hunting for decades.
“While it may not be as exciting to Douglas Adams fans, for mathematicians, finding a new solution for 3 is much more significant,” Andrew Sutherland, MIT mathematician, told Gizmodo.
The solution for the first non-trivial sum of three cubes that add to 3 is:
5699368212219623807203 + (-569936821113563493509)3 + (-472715493453327032)3 = 3
For decades, scientists have sought a’s, b’s, and c’s that satisfy the a3+b3+c3 = n equation, where n is a given integer. The number 3 has been a special example, though. While 1 and 2 have infinitely many solutions to this problem, based on a pattern, 3 only has two trivial solutions: 13+13+13 and 43+43+(-5)3.
In 1953, British mathematician Louis Mordell said it would be difficult to figure out if there are any others, and scientists went looking, unsuccessfully. Some even conjectured that no further solution existed.
Similar to the 42 announcement earlier this month, Sutherland and Andrew Booker of the University of Bristol found the answer using the Charity Engine, which lets scientists perform calculations with unused processing power from home computers.
The calculation took approximately 4 million computing hours, according to a press release from the university. Finding the solution was obviously difficult, but researchers were able to add an additional constraint to make the search faster: According to a previous proof, any answer requires the a, b, and c be a certain distance away from a multiple of nine.
As we’ve written, these sorts of problems are mainly interesting for cryptographic purposes. But from a mathematician’s perspective, they’re also just plain fun.
“For computational number theorists like me, having access to this kind of computational power is like giving an astronomer a new telescope that is 100 times more powerful than any that existed before,” Sutherland said. “There is no telling what you’ll see when you point it at what you thought was a dark patch of the sky.”