Mathematically speaking, the way a tiger charges a herd of antelope resembles a white blood cell attacking a colony of bacteria. That’s the conclusion of a new paper by an international team of physicists, just published in Journal of Physics A: Mathematical and Theoretical.
This, in turn, could shed light on the intricate dynamics of animal behaviour, as well as how bacterial invaders can evade attack and survive despite the best efforts of the immune system. “We would expect this to be a fairly good approximation for many animals,” lead author Ralf Metzler, of the University of Potsdam in Germany, said in a statement.
Predator/prey mathematical models are practically a workhorse in fields like ecology, biology and epidemiology, providing a handy means of studying how nature maintains an ecological balance. For example, members of the infamous Cordyceps family of fungi — over 400 different species in all — target various insect populations (usually ants, dragonflies, cockroaches, aphids or beetles).
The fungus scatters spores into the air, which land on a host’s body, working its way inside and sprouting long tendrils reaching into the host’s brain. Then it releases chemicals that turn the poor host into the fungi’s zombie slave. The fungus slowly consumes the brain, until it bursts through the host’s head, scattering even more spores into the air to infect more unsuspecting hosts. It’s pretty horrifying, especially for this poor bullet ant:
But Cordyceps serves an important purpose: controlling the populations of various pests. The dynamics play out something like this. If, say, an ant population grows so much that it threatens to overwhelm the available resources to sustain the colony, the fungus population will also start to increase, gradually thinning out the herd, so to speak. The ant population begins to diminish, meaning there are fewer hosts for the fungi to infect, so their numbers also decrease. Then the ants start multiplying, and the whole cycle starts all over again.
That’s one version of a predator/prey model. Metzler and his colleagues opted for a different version: that of a single predator hunting a herd of prey. Think of a lion or tiger chasing after an antelope herd, or a wolf charging a flock of sheep — or even a white blood cell homing in on a colony of bacteria.
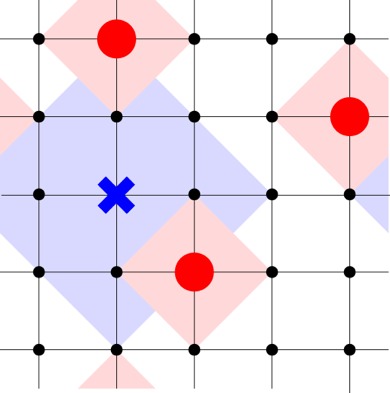
The predators are represented by the blue cross; the prey, by the red circles. Image: J. Phys. A: Maths. Theor. 49 225601
Their analysis revealed two different phases of the hunting process. At first, the predator’s motion is best described by a so-called random walk, as it searches for its prey. This involves searching a bit in one place, then moving to another likely spot nearby and searching there and so on. This is also a good foraging strategy for the prey.
Once the predator has spotted the prey, however, the dynamics shift to more of a ballistic trajectory: the tiger now wants to close the distance between itself and its prey as quickly as possible, while the prey wishes to evade the attack, beginning evasive manoeuvres in response.
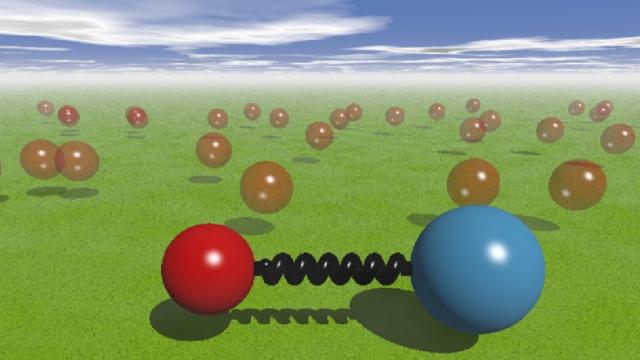
The researchers found that the charging tiger and the prey now move as if connected by a spring (see top image). You may have learned about Hooke’s Law in science class: the force needed to extend or compress a spring by a given distance is proportional to that distance. The tiger charges, the antelope begin to zigzag and scatter to evade the attack and, when the tiger finally closes the distance, an antelope falls.
Line of sight turns out to be pretty key to how those dynamics play out — the better the prey is able to spot encroaching predators, the better its chances of survival. So the physicists added this element to their model. Surprisingly, they found that the predator’s eyesight isn’t as critical. Predators benefit more from poor eyesight of the prey than from any improved vision of their own.
And sometimes especially large herds can get in each other’s way as they scramble to escape a charging predator. Other studies have found that herds can increase their survival odds by clustering in ways that optimise potential escape routes. Maybe that’s how certain bacterial colonies manage to survive a white blood cell’s attack.
It’s just one more example of the power of maths to tease out hidden connections between systems that seem on the surface to have nothing in common at all.
[Journal of Physics A: Mathematical and Theoretical]
Top: A charging tiger and its prey move as if connected by a spring. Image: Maria Schwarzl