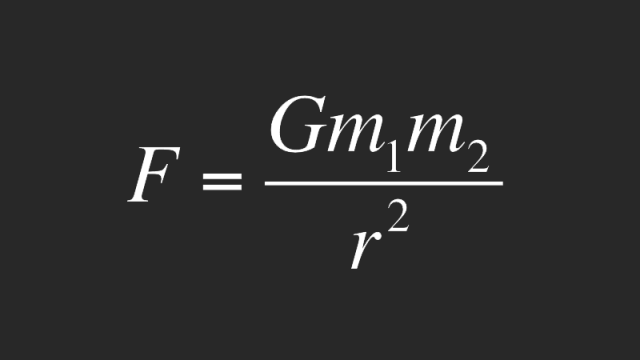What goes up must come down — though not always in the way you’d expect. This is Newton’s Universal Law of Gravitation, and you can thank it for GPS, Google Earth and even the pictures beamed back from the other side of the Solar System. Here’s why.
The equation neatly describes the gravitational attraction that two objects experience between each other, as a result of their masses and how far apart their centres lie. It’s this equation that predicts how hard objects fall to Earth when they’re dropped. But it wasn’t entirely Newton’s work, despite its name.
“The Power By Which The Sun Seizes Hold Of The Planets”
For centuries scientists pondered the motion of moons and planets in our Solar System, and many of them — fairly understandably — thought everything revolved around the Earth. It wasn’t until Johannes Kepler closely studied the motion of Mars in the early 17th Century that the idea of the planets orbiting the Sun really took hold. Even then, though, what Kepler did was generate three equations that described with impressive accuracy the elliptical motion of satellites in orbit — like the Moon around Earth, or Mars around the Sun. What he didn’t explain was how the central body held the other in its orbit.
Many a scientists tried to fill in the blanks. In 1645, the French astronomer Ismael Bouleau suggested that “the power by which the sun seizes hold of the planets… becomes weaker and attenuated at greater distances.” He was right, but his reasoning was dubious; he simply compared the attraction to rays of light. Later, in 1666, Robert Hooke took the same idea further, adding that “these attractive powers are so much the more powerful in operating by how much the nearer the body wrought upon is to their own Centres.” He was on the right track — but he still hadn’t nailed it.
In his 1687 publication of Philosophiæ Naturalis Principia Mathematica, though, Newton unleashed his Law of Gravitation upon the world. He brought together all that had gone before on the topic, formalising his thinking into the equation above. The equation allows us to calculate the size of the gravitational force, F, experienced by two objects as the product of their masses divided by the square of the distance between them, r, all multiplied by a gravitational constant, G. (Incidentally, that last number is measured experimentally. As of 2010, it took the value 6.67384 x 10−11 cubic meters per kilogram second squared; it will be updated in 2018.)
There were complaints that Newton was passing the work of others off as his own — most notably from Hooke. For what it’s worth, in a letter to Hooke in February 1676, Newton wrote that: “If I have seen further, it is by standing on the shoulders of giants.” While some scholars seem to think that it could have been a dig directed at Hooke’s physical stature — he was quite a small man — it’s perhaps more pleasant to assume that it was a rare flash of academic modesty from Newton.
The Apple
So how did Newton manage where others had failed? As the story goes, he was sat beneath an apple tree, when one of its fruits fell to Earth — and crucially, straight to Earth. Not sideways or diagonally, but straight down. He was in Lincolnshire, a county in the UK, at the time, but the same thing happened in Cambridge, in London — and everywhere else on the planet, for that matter.
What Newton had realised was that the gravitational force that others had described was pulling objects towards the center of the Earth. That, after all, was the only way the apple could fall to the ground the same way in England as in New England. And he also noted that the bigger the mass of the apple, the bigger the force attracting it downwards — and, correspondingly, the bigger the mass of the Earth, the bigger the force causing the attraction.
The realisation that the force acted toward the center of the objects was vital. Combined with the knowledge that the gravitational force dropped off with distance, it allowed Newton to multiply the mass of the two bodies then divide his equation through by the distance of separation squared. It makes sense: if you imagine that the gravitational field around an object is a series of concentric spheres all drawn about the center of the object, then the same gravitational field acts over a larger surface area as you move outwards. Given the surface increases with the square of the distance, r,then the gravitational force must decrease by the same ratio.
But as Newton mused on the that apple he began to think: what if the apple tree was bigger? Or much bigger? Or much, much, much bigger, so the apple was at the same height as the moon? Wait, why doesn’t the moon fall out of the sky like the apple does, given it’s not being held up by a tree?
The Moon Is Always Falling
The annoying answer to that question is that, in fact, it does. But unlike the apple, it also happens to moving sideways, too. Newton mused on this conundrum by thinking not about the Moon itself but a cannonball instead. Fire a cannonball horizontally from the top of your local hill, and it follows a curved path, moving horizontally through the sky but also downwards thanks to the gravitational attraction of the Earth. Fire it much faster, and it sweeps out a longer curve, but it still falls to Earth. Fire it just the right speed though — admittedly, a very high speed — and its horizontal speed is high enough that every time the cannonball drops towards Earth it’s also moved forwards far enough that the surface of the Earth has curved away underneath by the same amount.
That’s exactly what happens with the Moon. When that round lump of rock that orbits Earth was spawned, it gathered enough energy to provide it with the sideways motion to fly through space such that it never fell down to Earth, even though it was falling all the time. And with no other forces acting upon it — there ain’t no air resistance in space — it keeps going, and going, and going. This is, of course, what we now casually refer to as an orbit — and Newton’s Law of Gravitation allows scientists to work out how much of that sideways energy is required to put a satellite into that rotational path, rather than having it merely fall back to Earth like the apple.
It may be surprising but that single equation can give scientists enough information to loft satellites into orbit around our planet. All the satellites orbiting Earth — be they taking photographs, beaming positioning signals, or acting as home to astronauts — were put there with it. But analyse the motion of any of those satellites as they swing by Earth and the path doesn’t quite match up with the dynamics you’d expect based on the forces predicted by Newton’s equation. It’s not because his equation is wrong, though: it’s because each and every body in the Universe exerts a gravitational force on each and every other. Obviously the bigger and closer they are, the bigger the force — Newton exerted far less forces on that apple, for instance, than the Earth did — but that doesn’t mean every problem can be boiled down to simple systems of two bodies.
Newton’s Law, Plus Computers
The good news is that Newton’s Law of Gravitation is actually called Newton’s Universal Law of Gravitation. That means you can calculate all the forces exerted by different bodies, to work out which ones might actually matter. The bad news is that the maths required to calculate the resulting motion of the bodies based on all those forces isn’t the kind you can do with a pencil and paper. In fact, in 1890 when French mathematician Henri Poincaré tried to depict the resulting motion of three bodies — in his case a star, a planet and a dust particle — he said he was so “struck by the complexity of this figure that I am not even attempting to draw.” Oh dear.
Mercifully, along came computers — allowing the forces predicted by Netwon’s equation to be crunched in laborious detail why scientists sat around drinking tea. In fact, physicists giddy at the prospect of growing computational power realised that they could combine the forces generated by the biggest bodies in our Solar System to map out where gravity had the greatest effects — and, interestingly, where it cancelled out. Turns out that there are a series of interlinked tubes throughout space of constant — and crucially low — gravitational pull, that are now referred to as the Interplanetary Transport Network. Think of them as contours on a map that are easier to follow than walking uphill. Fire a craft through this network and they can navigate space with very little energy input — making it easy to travel a long way without much fuel.
In fact, these tubes are now used to send satellites to the far reaches of the Solar System. NASA’s Genesis and Solar and Heliospheric Observatory missions to the Sun used them; China sent its Chang’e 2 craft used to asteroid 4179 Toutatis along them, too. And that, along with the rise in computational power, is all thanks to Newton’s Law of Gravitation. Not bad for an apple.
This is the second in a new experimental series called Favoured Equation. Each month, we’ll dive into a piece of maths that makes your life easier in some way without you even realising it.