Perhaps the main difference if the earth were a cube would be that students would become much more frustrated trying to calculate the gravitational field.
For a uniform cube with side length L and density rho, the gravitational force on mass m at position (x,y,z) is given by
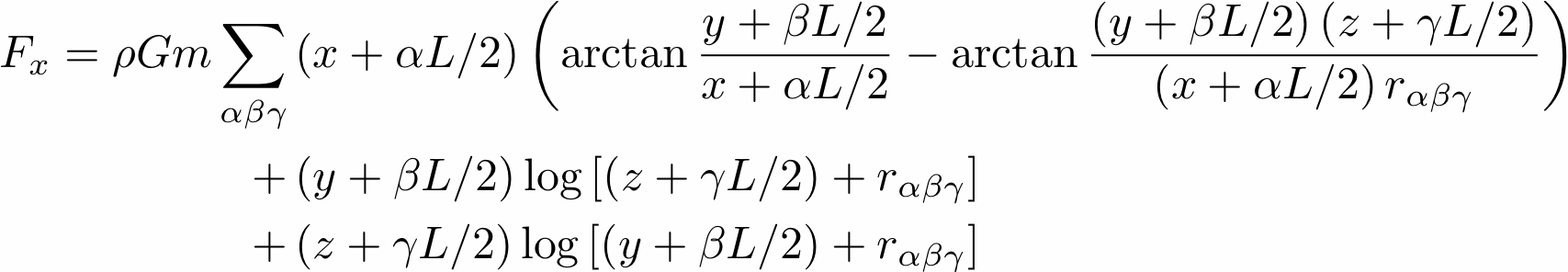
with
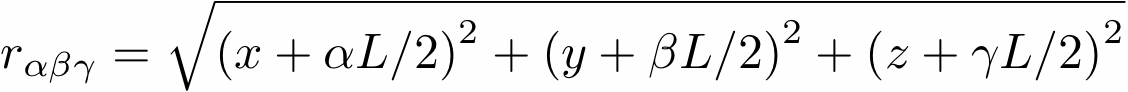
where alpha, beta, and gamma are +/-1. This is the x-component of the force. By symmetry, the y and z components are given by swapping y for x and z for x in the above equation. (It’s just a matter of integrating Newton’s law of gravitation over the cube, but it gets a little messy.)
If we want a cubical earth, with the same mass as spherical earth, we get L = 9340 km. Let’s set aside the concern that such a planet might not be stable for very long, and take a look at its properties.
The graphs below show lines of force in blue, and equipotential lines in purple for two different slices through the cube, as shown. The black square (rectangle) indicates the boundary of the cube.
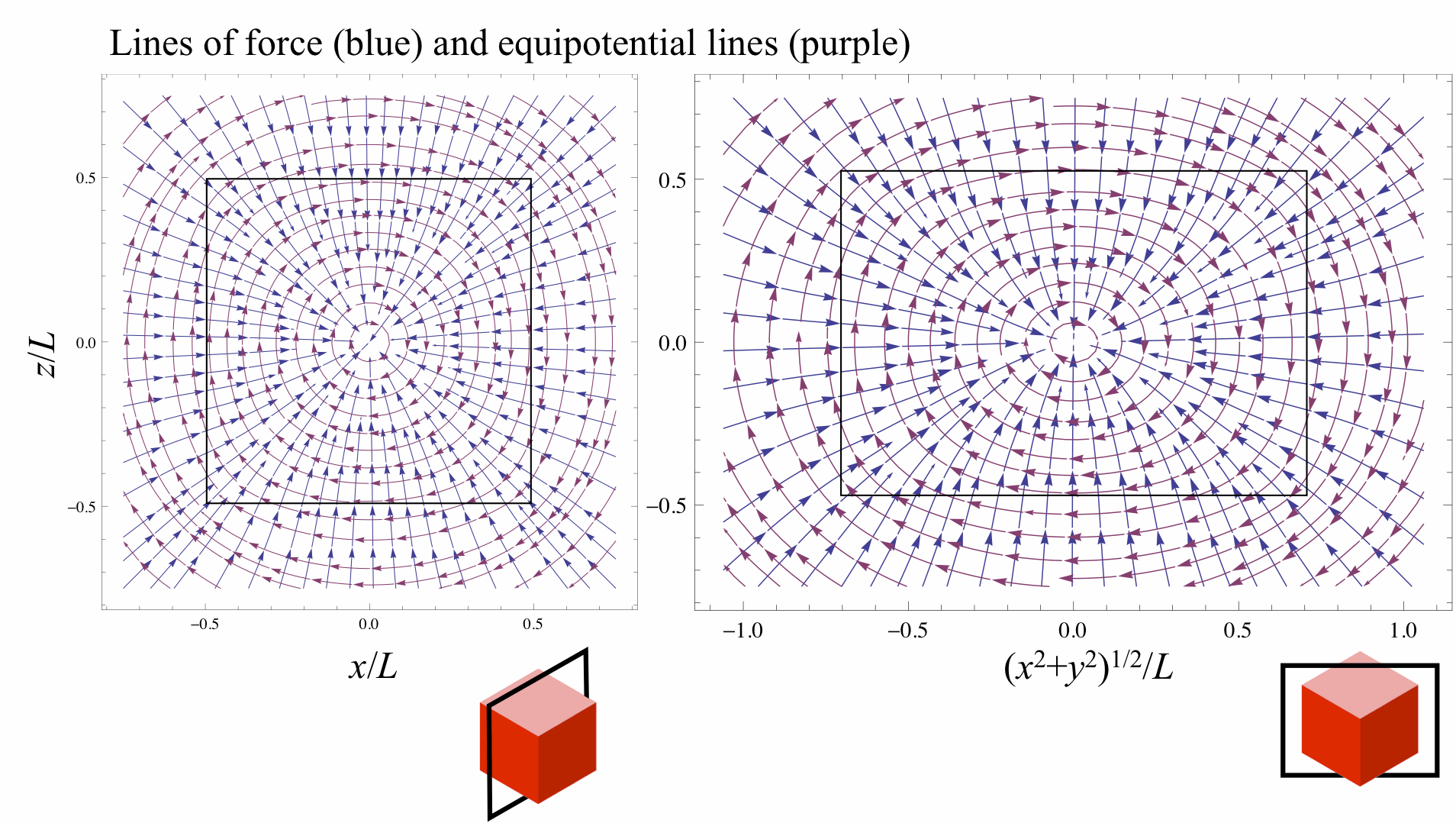
First, notice that at the surface of the cube, the lines of force are not in general perpendicular to the faces of the cube. If you were standing on the cube, you would therefore perceive that you were standing on a slope. The apparent angle of this slope is given by the angle of the gravitational force relative to the face of the cube. The graph below shows this perceived angle as a function of position over one face of the cube.
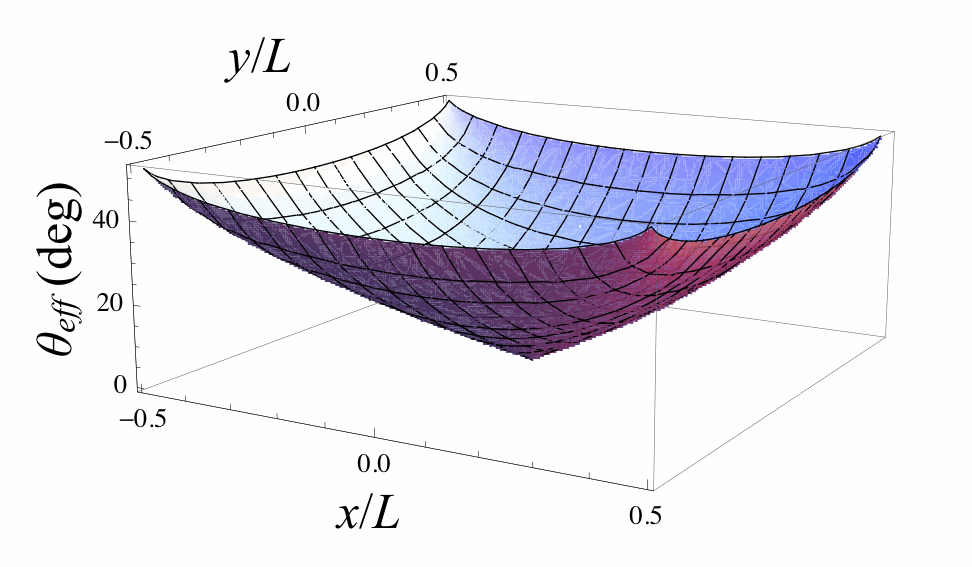
The apparent slope is higher near the corners of the cube, all the way up to about 50 degrees from the horizontal. Note that if you were standing on a face of the cube, you would not see the surface as shown in the figure above. The surface would appear completely flat, because it is. If you were accustomed to living on a spherical earth (as we are), I believe you would feel like the entire face of the cube you were standing on were sloped at a fixed angle, and this angle would depend on where on the face you were standing.
Second, we can take a look at the equipotential lines on the graphs above. These lines, shown in purple, are lines along which the gravitational potential energy is constant. If the earth’s water were divided equally among the faces of the cube, it would lie with its surface along an equipotential line. If we take the total volume of the water on earth (~1.4e9 km^3), I estimate that the six oceans would sit on each face, extending about L/5 from the center of the face, and having a height in the center of about 0.03L. This corresponds, perhaps, to the equipotential line closest to the face of the cube in the graphs above. That is, each ocean would be a lens shape with a round, somewhat squared off, perimeter. These lens shapes would be roughly 4000km across, and at the center, would extend about 300km up from the face of the cube.
The equipotential lines also indicate a line along which atmospheric pressure would be constant. From the right graph above, we can see that the atmospheric pressure at one of the corners is the same as the pressure about 0.22L (2200 km) above the center of the face. Seeing as how on spherical earth, the boundary between the atmosphere and space is considered to be somewhere around 100km, the same atmosphere on cubical earth would leave the corners of the cube quite far outside the atmosphere. In fact, if we were to choose a particular pressure as the boundary between atmosphere and space, this boundary would look similar to the lens shape described above for the oceans, but somewhat larger.
Another property of the cubical earth’s gravitational potential is that the force acting on you would decrease as you moved from the center of a face to a corner. The graph below shows the total force acting on a 100kg person as a function of position on a face of the cube, divided by the gravitational acceleration g at the surface of spherical earth. By dividing the force by g, we can see your “apparent mass” – the mass that would feel the same on spherical earth.
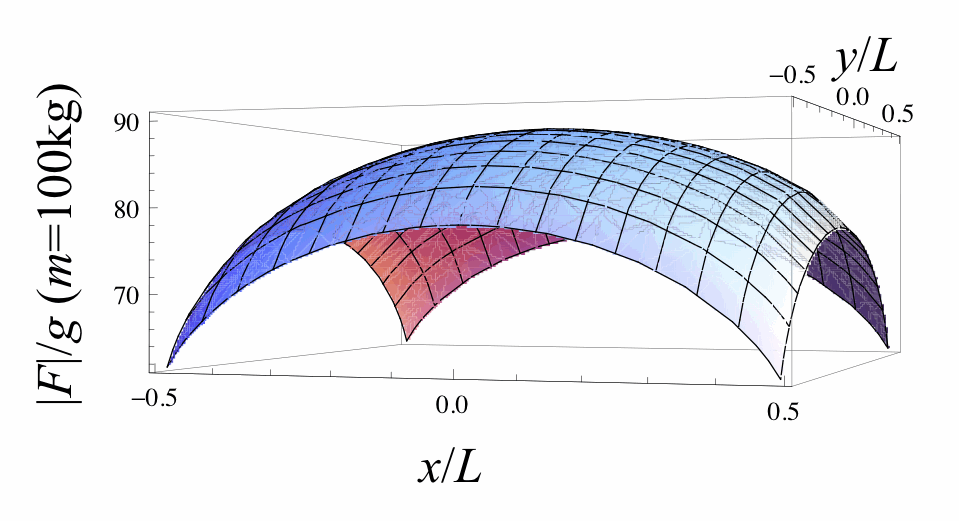
Note that even at the center of the face, you are lighter than on spherical earth, and your weight decreases significantly as you travel to the corners.
A final point of interest is to look at how the gravitational force scales with distance from the cube. On spherical earth, gravity decreases according to inverse square of distance from the center of the earth (F~1/r^2). The behaviour is more complicated for cubical earth. The graph below shows the power of r by which the gravitational force changes, along a line extending perpendicular from the center of one face.
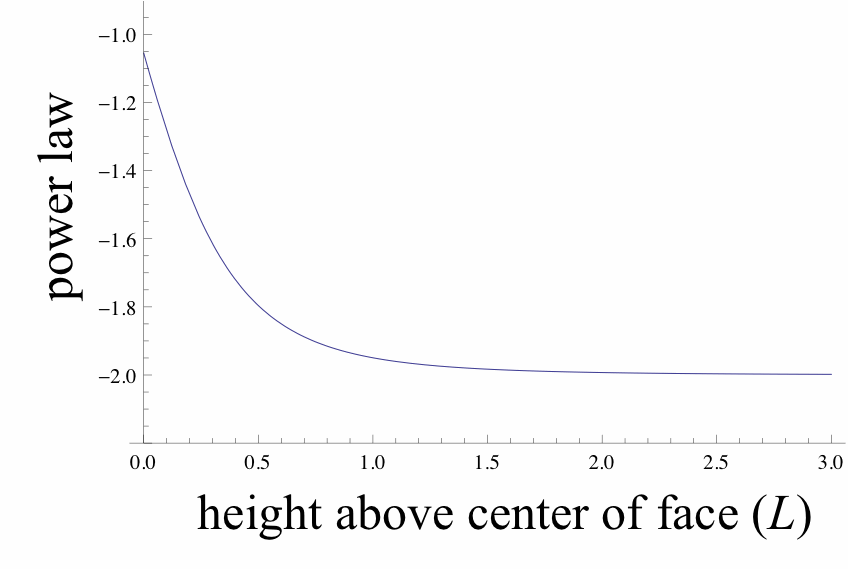
At large distances (>1 or 2L), the force falls off as 1/r^2. At this distance, the particular shape doesn’t really matter. But at closer distance, the force falls off more slowly, approaching 1/r near the surface.
What about equipotential surfaces, extent of oceans and atmospheres, and escape velocity?
I was unable to analytically find an expression for the gravitational potential of a cube, so I just had Mathematica numerically integrate the gravitational field along a path from the origin to the point of interest. From this, we can find quantitative values of the potential. Also, the equipotential lines can be evaluated numerically by solving a differential equation to find lines that are always perpendicular to the gravitational field.
The figure below shows two equipotential lines, where the vertical axis extend upwards from the center of a face, and the horizontal axis extends to the middle of an edge. This close to the surface, the equipotential surfaces are quite circular (see below), so the equipotential surface that contains this line can be approximated by revolving the line about the vertical axis. From this surface of revolution, we can calculate the volume contained between the cube and this surface. The purple line in the figure shows the approximate surface of the ocean, if the earth’s water were divided equally among the faces. If the atmosphere is such that pressure is 1 atm at the surface of the ocean, and we assume that pressure decreases exponentially (a pretty good approximation), then the boundary between “atmosphere” and “space” would be approximately at the blue equipotential line. (This boundary is chosen to be at the same pressure as at 100km above the spherical earth’s surface.) Interestingly, we see that the large majority of the cube’s surface is outside the atmosphere. Since humans can survive only at about 10km above the earth’s surface, this means that the habitable land on the cube would be a narrow ring around the oceans, about 10km wide.
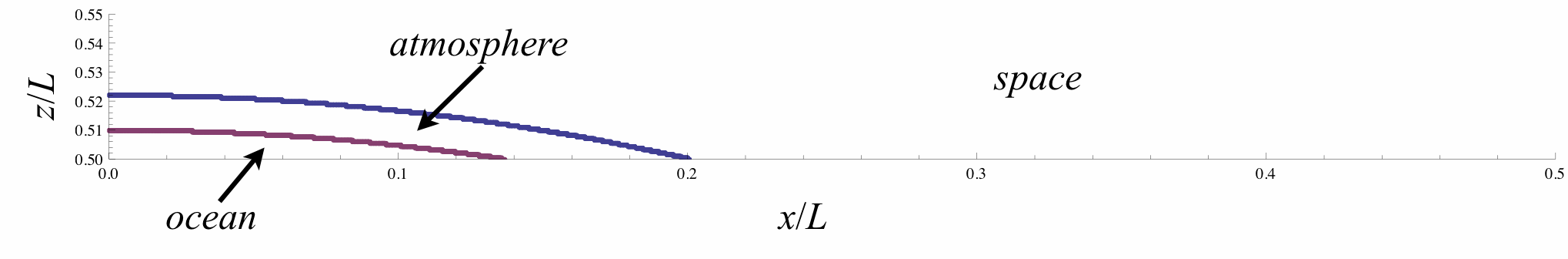
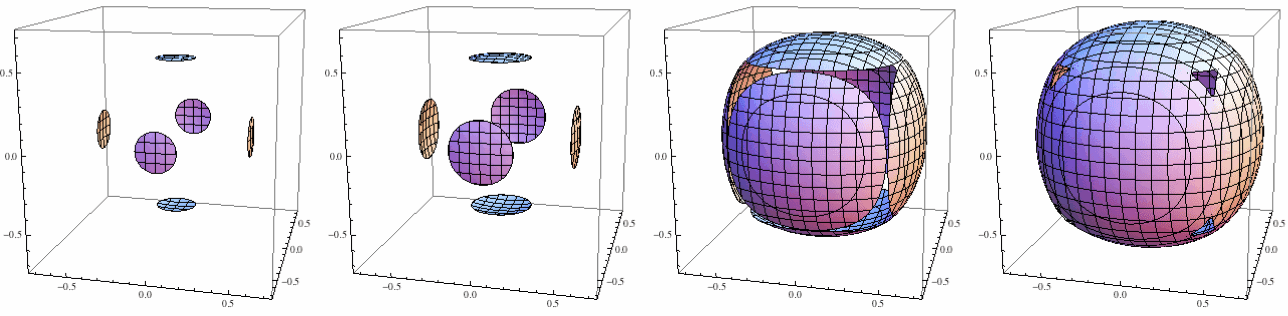
It is interesting to take a look at what the equipotential surfaces look like. The figure below shows 4 equipotential surfaces outside the cube at increasing values of the potential. The leftmost figure shows the shape of the oceans, and the second to left figure shows the boundary of the atmosphere, as described above.
We can now easily calculate the radius of curvature of the equipotential surfaces at any point. The figure below shows the radius of curvature R of the equipotential surface at a point a distance x from the center of the cube, going towards the center of a face. For comparison, the radius of curvature in a spherically symmetric potential is shown, with the particular value of the earth’s radius indicated.
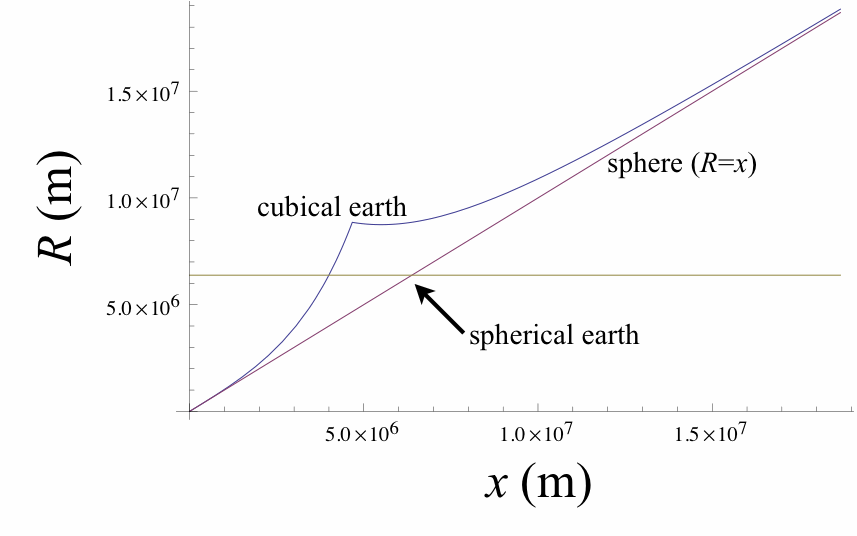
We see that when the equipotential surface is far from the surface of the cube, the curvature is about the same as if the mass were spherically symmetric. Near the surface (where we see the kink), the radius of curvature increases, meaning that the equipotential surface is flattened out, reflecting the shape of the cube.
In particular, the radius of curvature at the top of the ocean is about 8830 km, (For the surface of the oceans, we can assume that the surface is spherical, so the radius of curvature is the same everywhere.) Note that the top of the ocean is about 4770km from the center of the cube, so this is quite near the kink above. This radius of curvature is larger than the radius of curvature of the spherical earth (6400km). This results in a horizon that would be slightly farther away, as seen from the same height above the water. (At 10m above the surface of the ocean, the horizon is 13km away on the cube and 11km away on earth.) An interesting observation is that if you were sailing in a ship from the center of the ocean towards the shore, the first land to come in to view would be a very distant edge or vertex of the cube. A vertex could come into view in as little as 130 km from the center of the ocean. The shore would not come into view until it was over the horizon, about 1300km from the center of the ocean (and 13km from shore).
With the gravitational potential, we can also easily calculate the escape velocity – the velocity you would need to give to an object somewhere on the cube so that it will move away from the cube forever (neither fall back down nor go into orbit). This is just the velocity where kinetic energy is equal to the change in potential energy from the surface to infinity. At a vertex, escape velocity is about 6050 m/s. At the center of a face, it is about 7470 m/s. This can be compared to escape velocity on the surface of the earth, about 11000 m/s. If the cube is rotating with a period of 24 hours, the velocity of any point on the cube will be much less than escape velocity (which also the case for spherical earth). In the best case scenario, if the cube is rotating about an axis through the middle of two opposite edges, then farthest out point at one of the vertices is moving at about 600m/s.
Picture: Shutterstock/Anton Balazh
This post was republished with permission from Jesse Berezovsky, a Professor of Physics at Case Western Reserve University. It originally appeared on Quora.
