If there’s anything we learn from maths teachers and the Da Vinci Code, it’s that prime numbers are magic. They can do anything, and be anywhere. Including a doodle on a maths paper.
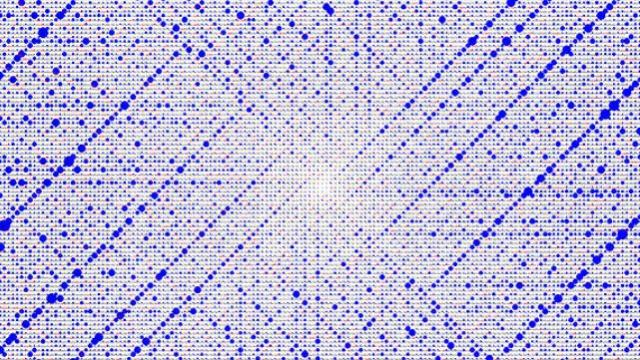
In the 1960s, a gentleman known as Stanislaw Ulam was making his way through a miserable meeting by doodling on a piece of paper. Unlike most of us, who only manage to do 3D cubes and obscene drawings of people we don’t like, Ulam tried filling his paper with maths. And he discovered something very strange. Ulam drew a ‘1’ at the centre of his paper. Directly to the right of the one he drew a ‘2’. Above the two he drew ‘3’, and continued spiralling the numbers outwards toward from the one. When he was done filling up the page, he decided to circle all the prime numbers – the numbers divisible only by one and themselves.
What he found was a lot of diagonal lines. They crisscrossed the paper, sometimes in short bursts and other times in long strings. While there are plenty of singularities and outliers, a large plot of the primes on Ulam’s Spiral shows a remarkable density of diagonals. Further plotting with computers show that these diagonals appear even when the numbers get high, and even when the spiral doesn’t originate with the number one. Change the spiral from one that’s plotted on a grid to one that’s plotted on a circular spiral, and the lines will change direction, but they’ll still be there. Plot it on the hexagon – more lines.
It’s things like this that make prime numbers so eery. They keep showing up in nature, in important functions, and in pure mathematical play. (I think they’re the ghosts of ancient Greek numerals.) [via Good Math]
Republished from io9